Mean is the average of the numbers in a data set. Take all the numbers in the set add them and divide the sum by the number of numbers that you have in your data set.

Median is easy to remember by thinking of the grassy area that divides both sides of the interstate. The median is the middle number. To find the middle number you must always put your set of numbers in order from least to greatest and then mark off numbers at both tails until you get to the middle. If there are two middle numbers after you have done the mark off step then you must average those two numbers to find the median. There will always be two middle numbers when you have an even number of data points in a set and there will always be one middle number when there is an odd number of data points in a set.

Mode is the number that appears the most. It is possible to have two modes or two numbers that appear exactly the same amount of times in a data set. If more than two numbers appear the same amount of times then the data is said to have "no mode".

Range is the greatest number minus the smallest number. It is the measure of the dispersion of the data set.

Interquartile Range is the dispersion of the data set between quartile one(Q1) and quartile three(Q3). To calculate interquartile range you must do the following steps.

1. First, find the median or middle of the data set.

2. Once you find the median of the entire set you must identify the lower and upper quartile.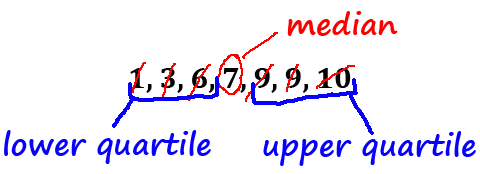

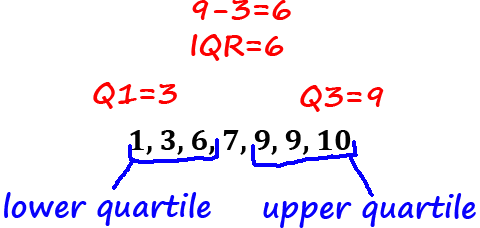
3. Now that you have identified your quartiles you need to find the median of the upper and the lower quartiles. The median of the upper quartile will be called Q3, while the median of the lower quartile will be called Q3.

4. Finally, to find the interquartile range you must subtract Q1 from Q3.

Population - A group of people or objects that you want information about.

Sample - A subset of a population.

Population Standard Deviation - The typical difference between a data value and the mean of a population. It is the measure of spread from the mean.

Sample Standard Deviation - The typical difference between a data value and the mean of a sample. Instead of dividing by the number of data points to find the mean you would divide by one less than the number of data points when calculating the standard deviation of a sample.

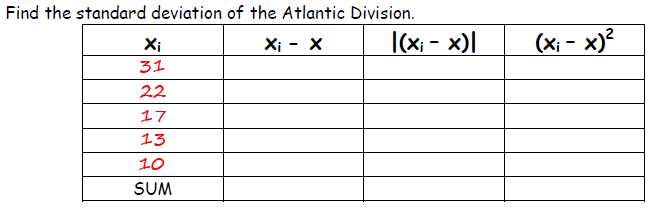
1. In the first column record all data points from greatest to least going down.

2. In the second column you are going to subtract the mean of the data set from each data point.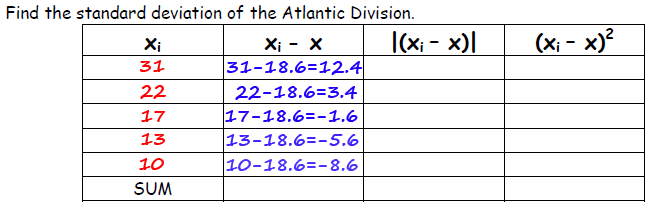

3. The third column is where you will write down the absolute value of each calculation from column two.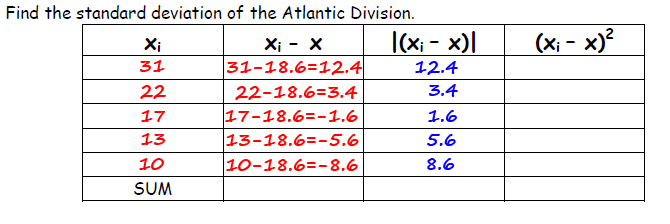

4. You will square each number from column 3 and record your answers in the final column.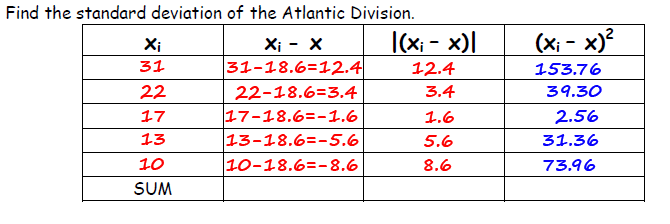

5. Take the sum of the 4th column.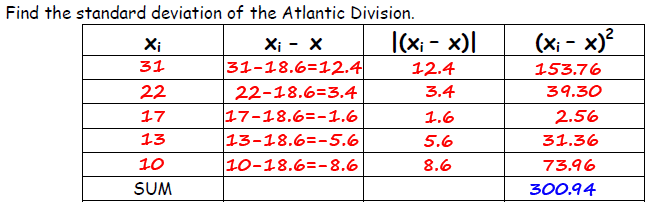

6. The final step in finding the standard deviation is to take the sum of the 4th column and squareroot it.

Credits

Images courtesy of Morguefile.com

Littell, McDougal,. McDougal Littell Georgia high school mathematics 2. 2008. Print.

Go to Top of Page